 Following the advent of powerful astronomical telescopes, observers saw objects much further out into space than had been previously possible. These newly-sighted objects looked redder than closer stars and galaxies that had been known for millennia. At first, it seemed that the bluer colours were being filtered out, more than were the redder ones, by the greater amount of interstellar medium through which light from these new more distant objects had to pass. But it was not that simple. This is because atomic emission and absorption lines within the light from these new more distant objects were also shifted towards the redder end of the visible spectrum. The wavelength of the light received must therefore necessarily have become greater than it was when it left its source.
Following the advent of powerful astronomical telescopes, observers saw objects much further out into space than had been previously possible. These newly-sighted objects looked redder than closer stars and galaxies that had been known for millennia. At first, it seemed that the bluer colours were being filtered out, more than were the redder ones, by the greater amount of interstellar medium through which light from these new more distant objects had to pass. But it was not that simple. This is because atomic emission and absorption lines within the light from these new more distant objects were also shifted towards the redder end of the visible spectrum. The wavelength of the light received must therefore necessarily have become greater than it was when it left its source.
This observed apparent reddening of light received from distant objects came to be known as red-shift. This signifies that, when the light they emit reaches us, it has suffered a reddening of its colour, which signifies an increase in its wavelength, which corresponds to a reduction in its frequency. What could be causing this red-shift? Before I can explain my answer to this question, it is first necessary to look a little deeper into my somewhat unconventional view of space and time.
The Observer's Event-Horizon
 Depicted within science's view of 4-dimensional space-time, all events, whose influence can affect me, at the moment we call now, must lie on the infinitely thin surface of the infinite red cone, as shown in the adjacent diagram. Such an event is indicated by the figure (1). An event inside the cone (2) happened too close to me for me to be affected by it now. Its influence passed by my present position sometime in the past and is currently travelling away from me. I may have been affected by it in the past, so long as its influence passed my location after I was born. An event outside the cone (3) happened too far away from me for its influence to have yet reached my current location. I could be affected by it in the future if I am still alive when its effect reaches me.
Depicted within science's view of 4-dimensional space-time, all events, whose influence can affect me, at the moment we call now, must lie on the infinitely thin surface of the infinite red cone, as shown in the adjacent diagram. Such an event is indicated by the figure (1). An event inside the cone (2) happened too close to me for me to be affected by it now. Its influence passed by my present position sometime in the past and is currently travelling away from me. I may have been affected by it in the past, so long as its influence passed my location after I was born. An event outside the cone (3) happened too far away from me for its influence to have yet reached my current location. I could be affected by it in the future if I am still alive when its effect reaches me.
The universe I see comprises events which took place within the sliver of space-time represented by the cone. This sliver of space-time is called my event-horizon. As my moment of now advances through time, the cone is dragged with it. Thus, at a moment δt beyond now, my visible universe will comprise a different sliver of space-time represented by an identical cone, whose apex is δt further into my future. It is easy to imagine a series of successive thin cones whose apexes are spaced δt apart in the direction of the future.
The conventional diagram above of an event-horizon reduces 3-dimensional space to a 2-dimensional plane, leaving the spare third dimension of space to represent time, which is presumed to be 1-dimensional. Thus, what is conventionally thought of as the 'now' event-horizon is represented by the surface of an infinite cone. This 3-dimensional representation is then projected perspectively on to a 2-dimensional sheet of paper or computer screen.
I do not like this representation. Space has 3 dimensions and cannot be conceptually represented correctly by a 2-dimensional plane. Furthermore, time is just as much a vector quantity as space. Related to space by a simple constant of proportionality, c, [the velocity of light], time has equal jurisdiction with space in all three dimensions.
A More Realistic Representation
This is why I prefer to represent the traditional notion of the relativistic event-horizon more pragmatically. That is, with 3-dimensional space represented by 3-dimensional space, as shown in the following diagrams.
 The diagram on the right shows the situation at an arbitrary instant called 'now'. The events E1, E2 and E3 occur simultaneously in time but at different distances from me. The radial in-flux of the æther brings information from each of these 3 events radially towards me at the velocity of light. I cannot see any evidence at this moment that event E1 ever occurred. This is because event E1 occurred too close to me. So all information about its occurrence will have passed the point where I am 'now' at some time in my past. I may have seen it in my past, assuming it passed by me after I was born. But I cannot see it now, nor will I ever see it in my future.
The diagram on the right shows the situation at an arbitrary instant called 'now'. The events E1, E2 and E3 occur simultaneously in time but at different distances from me. The radial in-flux of the æther brings information from each of these 3 events radially towards me at the velocity of light. I cannot see any evidence at this moment that event E1 ever occurred. This is because event E1 occurred too close to me. So all information about its occurrence will have passed the point where I am 'now' at some time in my past. I may have seen it in my past, assuming it passed by me after I was born. But I cannot see it now, nor will I ever see it in my future.
However, I am now witnessing the occurrence of event E2. This is because event E2 occurred at an instant in my past sufficiently far back for the information about it to have had just enough time to reach the point where I am now. The distance d from me, at which event E2 occurred, is how long ago t it occurred times the velocity of light c. In other words, d = t × c.
I cannot see any evidence at this moment that event E3 ever occurred. This is because event E3 occurred too far away from me. So all information about its occurrence has not yet reached the point where I am 'now'. I may be able to see it in my future, assuming it will pass by me before I die. But I cannot see it now, nor have I ever seen it in my past.
 The diagram on the left again shows the situation at an arbitrary instant called 'now'. Here, however, events E1, E2 and E3 occur at places that are equally distant from me but at different times. The radial in-flux of the æther brings information from each of these 3 events radially towards me at the velocity of light. I cannot see any evidence at this moment that event E1 ever occurred. This is because event E1 occurred too late [too close to me in time]. So all information about its occurrence will have passed the point where I am 'now' at some time in my past. I may have seen it in my past, assuming it passed by me after I was born. But I cannot see it now, nor will I ever see it in my future.
The diagram on the left again shows the situation at an arbitrary instant called 'now'. Here, however, events E1, E2 and E3 occur at places that are equally distant from me but at different times. The radial in-flux of the æther brings information from each of these 3 events radially towards me at the velocity of light. I cannot see any evidence at this moment that event E1 ever occurred. This is because event E1 occurred too late [too close to me in time]. So all information about its occurrence will have passed the point where I am 'now' at some time in my past. I may have seen it in my past, assuming it passed by me after I was born. But I cannot see it now, nor will I ever see it in my future.
However, I am now witnessing the occurrence of event E2. This is because event E2 occurred at an instant in my past sufficiently far back for the information about it to have had just enough time to reach the point where I am now. The distance d from me, at which event E2 occurred, is how long ago t it occurred times the velocity of light c. In other words, d = t × c. Same as before.
I cannot see any evidence at this moment that event E3 ever occurred. This is because event E3 occurred too early [too far back from my 'now']. So all information about its occurrence has not yet reached the point where I am 'now'. I may be able to see it in my future, assuming it will pass by me before I die. But I cannot see it now, nor have I ever seen it in my past.
 The universe that I see 'now' is illustrated in the diagram on the right. The condition that an event be included within my event-horizon is that information about it is just at the point of arriving at the place where I am now. Such events do not have to be simultaneous from my point of view, nor do they need to be equidistant from me. They each simply need to fulfil the condition: r = c × t, viz:
The universe that I see 'now' is illustrated in the diagram on the right. The condition that an event be included within my event-horizon is that information about it is just at the point of arriving at the place where I am now. Such events do not have to be simultaneous from my point of view, nor do they need to be equidistant from me. They each simply need to fulfil the condition: r = c × t, viz:
r1 = c × t1
r2 = c × t2
r3 = c × t3
where events E1, E2 and E3 occur amounts of time t1, t2 and t3 respectively back in my past; that is, before my 'now'.
Thus, my event-horizon in the above diagrams is the surface of a sphere, centred on me, whose radius demarcates the maximum distance from me that an event, which fulfils the condition r = c × t, can occur in order for it to be part of my 'now' universe.
The Scale of My Event-Horizon
Looking out into the universe, my 'now' event-horizon appears, for the most part, to be filled with galaxies, which tend to congregate into clusters. However, towards the extremity of my event-horizon — at a distance of about 11 billion light-years — is a shell of space, about 3 billion light-years thick, which appears to be populated by objects called quasars. Quasars do not seem to be stars or galaxies. They produce enormous power that has the standard distribution characteristic of synchrotron radiation.
Beyond the quasars, the feature that appears to be the furthest from me in my 'now' event-horizon is a phenomenon known as the Cosmic Microwave Background. The Cosmic Microwave Background thus marks the maximum extent [or radius] of my 'now' event-horizon. Its source is currently estimated to be 13·82 billion light-years distant.
 In the diagram on the left, the small central blue sphere represents the limit at which stellar distances can be determined geometrically to an accuracy of ±10%. Its radius is about 4 millimetres on my computer screen. On this same scale, the brown circle representing the source of the Cosmic Microwave Background should really have a radius of 110 kilometres. In other words, almost 28 million times the maximum distance at which it is possible to measure stellar distances accurately by parallax. Can it be safe to assume that, what appears to be ostensibly linear throughout its range of measurability, will remain so when it is extrapolated to 28 million times that range?
In the diagram on the left, the small central blue sphere represents the limit at which stellar distances can be determined geometrically to an accuracy of ±10%. Its radius is about 4 millimetres on my computer screen. On this same scale, the brown circle representing the source of the Cosmic Microwave Background should really have a radius of 110 kilometres. In other words, almost 28 million times the maximum distance at which it is possible to measure stellar distances accurately by parallax. Can it be safe to assume that, what appears to be ostensibly linear throughout its range of measurability, will remain so when it is extrapolated to 28 million times that range?
Personally, I think it is a very unsafe assumption. It looks to me like a vain attempt to rescue an untenable hypothesis. Relationships in nature are rarely linear. The laws of physics are replete with non-linearity, however slight it may appear over limited ranges. So, is it safe to assume that the apparent linear correlation between cosmic distance and red-shift remains linear when it is extrapolated almost 28 million times beyond the limit of accurate geometrical distance measurement? I think not.
 To get a sense of scale here, let us consider our own local galaxy, the Milky Way. We are inside the Milky Way galaxy, located approximately where the red circle is as shown on the right. The diameter of our galaxy is estimated to be from 100 to 180 thousand light-years. Let us suppose it has a radius of 70,000 light-years. The maximum range out to which we can measure the distance of an object accurately by geometrical parallax is about 500 light-years. We can therefore only estimate the distance of a stellar object accurately up to 500 ÷ 70,000 ≅ 0·007 of the radius of our own galaxy. That is as far out as the radius of the white dot inside the red circle shown in the illustration.
To get a sense of scale here, let us consider our own local galaxy, the Milky Way. We are inside the Milky Way galaxy, located approximately where the red circle is as shown on the right. The diameter of our galaxy is estimated to be from 100 to 180 thousand light-years. Let us suppose it has a radius of 70,000 light-years. The maximum range out to which we can measure the distance of an object accurately by geometrical parallax is about 500 light-years. We can therefore only estimate the distance of a stellar object accurately up to 500 ÷ 70,000 ≅ 0·007 of the radius of our own galaxy. That is as far out as the radius of the white dot inside the red circle shown in the illustration.
Notwithstanding, in order to measure stellar distances by parallax, it is necessary to have a standard base distance that is accurately known by other means. The base distance used is the radius of the Earth's orbit around the sun. This is known as the Astronomical Unit. This was historically determined by using two observatories separated by a known distance on the ground. This known distance should be as large as possible, preferably the full diameter of the Earth. This method suffers from amplified error because the base distance is so small compared with the distance being measured. Nowadays, radar is used to measure distances within the Solar System. Nevertheless, this too suffers, albeit to a lesser extent, from the amplified error of extrapolation. Nonetheless, this baseline for measuring distance by parallax is itself an extrapolation and therefore of limited accuracy.
But even with this baseline, it is only possible to make any sense of stellar distances as far out as the outer rim of the white dot within the red circle in the above illustration. To measure distances on the scale of the Milky Way galaxy and beyond, it is necessary to devise a ladder of further methods, which can again only be extrapolations based on the parallax method. These methods determine distance as a function of the brightness of various types of cosmic object such as fluctuating stars, supernovae, globular clusters and galaxies. But even in theory, these methods are subject to inaccuracies from 25% to 40%. But this assumes that the interstellar medium through which the light from these objects travels does not attenuate or disperse their light in any hitherto unknown way. Consequently, the measurement of distance on cosmic scales is, at the very least, extremely tenuous.
Yet these are the only means available for determining any direct correlation between red-shift and distance. Nonetheless, the correlation between the distance and the red-shift of a luminous object is assumed to be established. How?
The Prime Axiom
To answer this, I need to go all the way back to the act of faith, that is the foundation upon which the whole of physics is built. It is known as the Prime Axiom. It is the blind assumption that the true Laws of Physics are exactly the same in all places at all times. In other words, they are immutable: being independent of space and time.
The Prime Axiom thereby forces me to conclude that the atoms within the distant quasi-stellar objects at the extremity of my vast event-horizon behave in exactly the same way — obey exactly the same laws — as do the atoms in any physics laboratory here on Earth. It further implies that all the known universal physical constants have exactly the same values there as they do here.
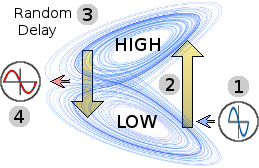 Here on Earth, an atom of a particular type [let’s say it's hydrogen], when provoked in some way, absorbs (1) a naturally-determined quantity of 'electromagnetic' energy of a narrow naturally determined wavelength. This jolts the atom (2) up to one of its HIGH energy states. This narrow naturally determined wavelength, at which the atom absorbs this 'electromagnetic' energy, is called an absorption line.
Here on Earth, an atom of a particular type [let’s say it's hydrogen], when provoked in some way, absorbs (1) a naturally-determined quantity of 'electromagnetic' energy of a narrow naturally determined wavelength. This jolts the atom (2) up to one of its HIGH energy states. This narrow naturally determined wavelength, at which the atom absorbs this 'electromagnetic' energy, is called an absorption line.
After what seems to be a random amount of time (3), the atom spontaneously falls back to its former LOW energy state, releasing a naturally-determined quantity of 'electromagnetic' energy (4), which is of a naturally determined longer wavelength. This narrow naturally determined wavelength, at which the atom [re]emits this 'electromagnetic' energy, is called an emission line.
The absorption and emission lines for a particular type of atom are referred to collectively as its spectral lines.
The times and wavelengths in this process are determined by the nature of the hydrogen atom. Consequently, they are the same for all hydrogen atoms wherever they may be. They are the same for a hydrogen atom in a terrestrial laboratory as they are for a hydrogen atom in the middle of a quasi-stellar object at the extremity of my event-horizon.
The hydrogen atom has many different spectral lines. These occur over a large proportion of the 'electromagnetic' spectrum from the ultraviolet to the hydrogen radio line with a wavelength of 21cm [corresponding to a frequency of 1420 MHz].
 The emission lines of hydrogen that fall within the visible spectrum are illustrated on the right. Their relative spacings are a unique signature showing that hydrogen is present in a source of light.
The emission lines of hydrogen that fall within the visible spectrum are illustrated on the right. Their relative spacings are a unique signature showing that hydrogen is present in a source of light.
If the Prime Axiom be true, then we may safely assume that the relative spacings of these lines and their absolute positions within the 'electromagnetic' spectrum must be the same for a hydrogen atom in a distant quasi-stellar object as they are for a hydrogen atom in a terrestrial laboratory.
Not What is Observed
But this is not the way it appears when we observe distant objects through an astronomical telescope. Distant objects appear redder than would be expected. However, this can neither confirm nor deny that the light originating from the distant object has, in itself, become redder. After all, how can we know that the colour has shifted? Why shouldn't the original colour of the galaxy simply be the colour we see it?
 Or perhaps the interstellar medium, through which the light has passed, attenuates or disperses the shorter [bluer] wavelengths much more than the longer [redder] wavelengths (see illustration).
Or perhaps the interstellar medium, through which the light has passed, attenuates or disperses the shorter [bluer] wavelengths much more than the longer [redder] wavelengths (see illustration).
Free space has what is known as an 'electromagnetic' reactance, X0 = μ0 ÷ ε0, its magnetic permeability divided by its electrical permittivity. But it also has a resistance R0, which is far from constant. Thus free space has an impedance, Z0, which is the vector sum of its reactance, X0 and its resistance, R0, Free space must therefore absorb and dissipate — to some very small extent — the energy of any 'electromagnetic' wave passing through it. Dust also disperses 'electromagnetic' radiation to a different degree in different places, more so the shorter the wavelength. This results in more distant objects appearing redder because the green light is attenuated more and the blue light even more still.
Notwithstanding, we also see that the absolute position of the characteristic pattern of an atom's spectral lines has been shifted to the redder end of the electromagnetic spectrum. The wavelength of each line has become significantly longer. So both its frequency and its energy have decreased. Consequently, red-shift cannot be explained as attenuation or dispersion caused by the interstellar medium because this would merely reduce the amplitudes of the bluer colours relative to the redder colours. It would not shift the wavelengths or frequencies of the spectral lines.
The following diagram illustrates red-shift for the emission lines of hydrogen. The upper pattern is obtained by passing light from a laboratory hydrogen discharge lamp into the upper half of a spectrometer's prism. The lower pattern is obtained by passing light from an astronomical telescope trained on a distant cosmic object into the lower half of the same spectrometer's prism.
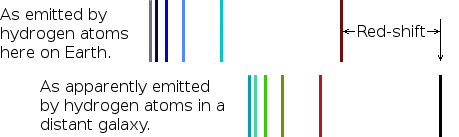
Other types of atom, such as magnesium and calcium, provide different characteristic patterns. These are also used for measuring red-shift.
Red-shift is expressed as the ratio, z, between the red-shift in the wavelength, Δλ [the observed wavelength, λobs, minus the reference wavelength, λref] divided by the reference wavelength, λref.
| z = |
λobs
- λref
λref
| = | Δλ
λref
|
The precariousness of the methods of measuring distance notwithstanding, the relationship between the distance of the object and its red-shift appear to have a linear relationship where 0 < z < 0·1. For values of z > 0·1, the relationship converges or diverges, according to which one of the currently accepted theories is applied. Red-shifts for the most distant known galaxies and quasars [from 11 to 12·9 billion light-years] is between about 7 and 8½. The greatest known red-shift of 1100 is that of the Cosmic Microwave Background, at a distance of 13·82 billion light-years. From these figures it is clear that, at the outer reaches of our event-horizon, the relationship between red-shift and supposed distance is extremely non-linear.
The Cause of Red-Shift
From the above, we could reasonably deduce that red-shift is simply caused by plain simple distance: the amount of space [or interstellar medium] through which the light has passed, however non-linear the relationship may appear to be at the extremity of our observable universe. But this is not the mainstream belief.
The best known phenomenon, which causes a change in the wavelength [and hence the frequency] of an 'electromagnetic' wave during its journey from its source to an observer, is the Doppler effect. The Doppler effect is apparent when the observer and the source are either approaching or receding from each other. When they are approaching each other, the wavelength of the 'electromagnetic' radiation received by the observer is shorter than the wavelength of the 'electromagnetic' radiation 'emitted' by the source. When they are receding from each other, the wavelength of the 'electromagnetic' radiation received by the observer is longer. An increase in wavelength corresponds to a decrease in frequency and vice versa.
The Doppler effect also occurs with other kinds of waves such as sound waves. However, the mechanism is somewhat different because it can involve the motion of the medium through which the waves travel, which does not apply to 'electromagnetic' radiation.
Because it fits the bill, the Doppler effect is thought to be the cause of the red-shift in the light arriving from distant galaxies and quasars. This implies that these distant objects must be receding from us at very high velocities. This implies that the universe is expanding or, more correctly, that the objects within it are dispersing: moving away from each other. Thus the velocity with which a galaxy recedes is a positive function of its distance from the observer.
For 'electromagnetic' radiation, the velocity with which a luminous object is receding from an observer is given by the formula: v = c × Δf ÷ f, where c is the velocity of the wave, Δf is the red-shift in the frequency of a reference line within the spectrum pattern the observer sees in his telescope and f is the frequency of the same reference line as originally emitted by the luminous object, which is assumed to be the same as that produced by a local hydrogen discharge lamp in the laboratory.
In using a spectrometer, it is more convenient to work with wavelength rather than frequency. In this case, the velocity with which a luminous object is receding from an observer is given by the formula: v = c × Δλ ÷ λ, where c is the velocity of the wave, Δλ is the red-shift in the wavelength of a reference line within the spectrum pattern the observer sees in his telescope and λ is the wavelength of the same reference line, again, as seen by the observer in his telescope.
Using the precarious ladder of cosmic distance measurement, astronomers calculate that objects are receding from us at a velocity, v, that is proportional to their distance d from us. Thus, v = H × d, where H is the necessary constant of proportionality, which is known as "Hubble's constant". Current calculations put the value of H at from 15 to 30 km/s per million light-years of distance. The reciprocal of Hubble's constant is thus the age of the universe, which works out at between 10 and 20 billion years.
Notwithstanding, the idea of the Doppler effect being the cause of red-shift is problematic. This is because the observed red-shifts of some galaxies puts their velocities of recession at around 2½ times that of light. If this were true then the light from those galaxies could never reach us. We could not possibly see them. They would be well and truly outside our event-horizon. But we do see them. Consequently, they must be within our current event-horizon.
Expanding Space-Time
To the best of my understanding, cosmologists believe that the red-shift in the light originating from distant cosmological objects is indeed a result of the Doppler Effect. But they also believe that the velocity of light, c, is constant throughout the universe, and that nothing can travel faster than this. Yet the amount of red-shift observed, when interpreted as a Doppler shift, corresponds to a rate of recession of (2½)c for these most distant cosmological objects and 3·2c for the spherical surface-of-origin of the Cosmic Microwave Background.
To overcome this apparent paradox, cosmologists postulate that these very distant galaxies are not really travelling away from us faster than light but that the observed red-shift is due to the fanciful fact that the space between them and us is itself in a continual state of expansion. Thus, as the light travels from its origin to the observer, it is propagating through continually expanding space. The consequence is that, as it travels, its waves become increasingly stretched: they become longer. By the time they reach the observer, waves that corresponded to yellow light when they left their source have become red: they have shifted towards the redder end of the spectrum.
I think it is universally self-evident that space-time is not nothing. It has real and measurable fundamental attributes. For example, it has an electrical permittivity, ε0, and a magnetic permeability, μ0, which is said to cause 'electromagnetic' waves to propagate through it at a velocity of c, where:
| c =
| 1
ε0×μ0
| = 299792458 metres per second.
|
Some scientists believe that it also has an ultimate granularity: a smallest possible indivisible distance of 1·6...×10-35 metre [called the Planck Length] and a smallest indivisible interval of time of 5·39...×10−44 second [called the Planck Time], whereby:
| c =
| Planck Length
Planck Time
| =
| 1·6... × 10-35
5·39... × 10−44
| = 299792458 metres per second.
|
If there exists an absolute minimum meaningful period of time [the Planck Time], then it must be the period of time within which the most fundamental natural finite-state mechanism can flip from one state to another. This, in turn, must set the transition times with which larger natural mechanisms, such as atoms, can change from one complex dynamical state to another. This, in turn, must determine the ramp times [and hence frequencies] and burst durations of the sets of discrete electromagnetic emissions [spectral lines] they produce.
As a quick aside, it also suggests to me that the Planck Time must be the absolute minimum periodicity that a wave can have. The highest possible frequency must therefore be 1·85528757×1043 Hz. Notwithstanding, the Planck Length and Planck Time are precepts of theories that follow the current vogue of viewing everything through the eyes of digital technology. I do not think that nature is digital in the sense of the granulation of space, time and all that is therein. Digitization is more to do with the way in which the human mind represents nature symbolically rather than with nature's inherent dynamical structure.
So space-time has real measurable absolute characteristics. One could say therefore that it has an essence or even substance. I think it reasonable to conclude from this that the substance of all things that are within space-time — from fundamental particles to galactic super-clusters — is that of space-time itself. I would go so far as to say that all objects — from fundamental particles upwards — are simply complex dynamical convolutions within the essence of space-time.
Consequently, whatever may affect space-time, must also affect everything that is of it and within it. So, if space-time itself be expanding or contracting, then everything that is of it and within it must also be expanding or contracting respectively. That is, all fundamental particles, atoms, humans, planets, stars, galaxies, clusters and super-clusters must be themselves all expanding or contracting along with the space-time that contains them and is the essence of their substance.
 A hydrogen atom at the far reaches of my event-horizon spontaneously emits a pulse of green light [as arrowed in the adjacent diagram]. About 12 billion years later, I see that event here on Earth.
A hydrogen atom at the far reaches of my event-horizon spontaneously emits a pulse of green light [as arrowed in the adjacent diagram]. About 12 billion years later, I see that event here on Earth.
If space-time be expanding, then, from my point of view, the Planck Length is shorter in the vicinity of that hydrogen atom. To preserve the invariance of c, the Planck Time there must be correspondingly shorter. Thus, from my point of view, that hydrogen atom is smaller than a hydrogen atom that is local to me. Furthermore, it operates faster. So the pulse of light it emitted must, from my point of view, be of a shorter wavelength than that of the corresponding emission line of a local hydrogen atom. In other words, it is effectively blue-shifted at the point of emission. The intervening space between that distant hydrogen atom and me [the observer] is expanding. This causes a Doppler red-shift in the pulse of light as it travels across the intervening space. And red-shift is what I see. I see the light from that distant hydrogen atom arriving at my eyes as having been red-shifted. Consequently, the Doppler red-shift must be so large that it over-compensates for the blue-shift caused by the faster atomic emission process in the distant hydrogen atom itself, leaving the visible red-shift that I see.
Expanding space-time is problematic. It is too complicated. When interpreted as a Doppler effect, red-shift shows very distant objects and clusters of objects to be receding. However, objects within galactic clusters do not seem to be dispersing as would be expected if space were expanding. Thus it would appear that only inter-cluster space is expanding, while intra-cluster space is not. But if space itself be expanding, then one would expect that expansion to be uniform and homogeneous. To resolve this anomaly, cosmologists postulate that the would-be dispersion of objects within a cluster is countered by extra gravitational attraction supplied by an intra-cluster 3-dimensional web of what they call dark matter. Thus the expansion of space is only apparent outside a cluster where dark matter is assumed to be sparse or non-existent. Again, when interpreted as a Doppler effect, red-shift shows the most distant objects to be receding at 2½ times the velocity of light, with the spherical surface of original emission of the Cosmic Microwave Background receding at about 3·2c [amazingly close to πc]. Furthermore, it seems that, according to the calculations of cosmologists, the rate at which space is expanding is actively increasing. This necessitates an on-going supply of energy for which there is no apparent source. To resolve this anomaly, cosmologists postulate that the universe is permeated by an undetectable reservoir of energy they refer to as dark energy.
To construct a theory of the universe based on expanding space-time, cosmologists therefore have to balance a succession of three unverifiable postulations upon an assumption about an observation, viz:
| OBSERVATION | Red-shift in the light from remote cosmic objects
|
|---|
| ASSUMED | to be a result of the Doppler effect
|
| ANOMALY 1 | shows remote galaxies to be receding at 2·5c
|
| POSTULATE | that space-time itself must be expanding
|
| ANOMALY 2 | intra-cluster space does not seem to be expanding
|
| POSTULATE | dark matter to counter local expansion of space
|
| ANOMALY 3 | space would have to expand at an accelerating rate
|
| POSTULATE | dark energy to power the acceleration
|
If space-time be contracting, then, from my point of view, the Planck Length is longer in the vicinity of that distant hydrogen atom. To preserve the invariance of c, the Planck Time there must be correspondingly greater. Thus, from my point of view, that hydrogen atom is larger than a hydrogen atom that is local to me. Furthermore, it operates slower. So the pulse of light it emitted must, from my point of view, be of a longer wavelength than that of the corresponding emission line of a local hydrogen atom. In other words, it is effectively red-shifted at the point of emission. The intervening space between that distant hydrogen atom and me [the observer] is contracting. This causes a Doppler blue-shift in the pulse of light as it travels across the intervening space. But I see a red-shift. I see the light from that distant hydrogen atom arriving at my eyes as having been red-shifted. Consequently, the Doppler blue-shift must be too small to compensate fully for the red-shift caused by the slower atomic emission process in the distant hydrogen atom itself, thus leaving the visible red-shift that I see.
If space-time be contracting, then the rate of contraction involved must be very small compared with the rate of expansion in the case of expanding space-time. This is because contracting space-time produces a blue-shift, whereas we see a red-shift. To over-compensate for this transital blue-shift, the red-shift resulting from the larger slower atoms emitting longer waves would require an enormously greater lengthening of Planck Length and Planck Time than the shortening required in the case of expanding space-time. Our local space must therefore be proportionally much more contracted compared with the space near the edge of our event-horizon than it would be expanded in the case of expanding space. Hence the distances of the most distant objects must be stupendously greater in this case, which would probably put their velocities at hundreds of times the velocity of light. This makes contracting space-time even more problematic than expanding space-time.
And it is likewise based on the same precarious balancing of 3 layers of postulation upon an assumption about an observation.
If space-time be static, that is, neither expanding nor contracting, then, from my point of view, the Planck Length must be the same in the vicinity of that distant hydrogen atom as it is in my locality. And to preserve the invariance of c, so must be the Planck Time. Thus, from my point of view, that hydrogen atom is the same size as a hydrogen atom that is local to me. Furthermore, it operates at the same speed. So the pulse of light it emitted must, from my point of view, be of the same wavelength as that of the corresponding emission line of a local hydrogen atom. In other words, it is neither red-shifted nor blue-shifted at its point of emission. The intervening space between that distant hydrogen atom and me [the observer] is static: it is neither expanding nor contracting. Thus there can be no Doppler shift in the wavelength of light as it travels across the intervening space. But I see a red-shift. I see the light from that distant hydrogen atom arriving at my eyes as having been red-shifted. Consequently, this red-shift cannot be due to the Doppler effect of expanding space. If indeed it be due to the Doppler Effect, then that Doppler Effect has to have resulted from the velocity with which that hydrogen atom is receding from me while travelling through space. Notwithstanding, the amount of red-shift that I see places the velocity with which the distant hydrogen atom is receding at about 2·5c, in which case, the light would never have reached me.
The fact that we can observe — and, theoretically, be gravitationally affected by these remote red-shifted galaxies — means that they cannot be receding from us faster than the light that has managed to reach us from them. So the red-shift that I see can't be a result of the Doppler Effect. It must have been caused by a different phenomenon.
A further prospective candidate, as a theory for the cause of red-shift, is the notion that the universe could have the form and behaviour of a 3-dimensional hurricane.
A hurricane has the form of a swirling [rotating] mass of clouds, comprising zillions of water molecules. However, the angular velocity of rotation, within the hurricane, is not constant. It diminishes with increasing radius, with discrete 'circular' bands of cloud each moving at its own particular angular velocity. Thus, extra turbulence is created between adjacent layers by the sheering effect caused by these differences in angular velocity.
Galaxies clearly appear to have this same complex-dynamical form and behaviour, although, like a hurricane, their motion is restricted ostensibly to just 2 dimensions. If the universe be composed of galaxies, it could be seen as having the form and behaviour of a gigantic 3-dimensional weather system. I would not attempt to predict what kind of effects the resulting gravitational sheering and irregular angular shifts would have on the passage of light across such a tempestuous cosmos.
The Simplest Option
I see the light arriving from a distant galaxy as red-shifted. Notwithstanding, if the Prime Axiom be true, then the distant space-time in which that galaxy is located, can be neither compressed nor expanded relative to my local space-time. The values of such universal constants as the Planck length [lp] the Planck time [tp] the electrical permittivity of free space [ε0] and the magnetic permeability of free space [μ0] must all have exactly the same local values in both places and epochs. They are invariants: they cannot change over time and space. Consequently, an alien observer [today] in that distant galaxy must see the light from the Milky Way galaxy [as it was 12 billion years ago] red-shifted by the same amount.
If space-time be in a continual state of expansion or contraction, then so too must be the above invariants. The Prime Axiom is thereby violated. If, on the other hand, space-time be neither expanding nor contracting, then, when interpreted as a Doppler effect, the observed red-shift indicates that the galaxy must be receding at about 2·5c. The Prime Axiom is again violated. If we concede that the Prime Axiom indeed be false, then red-shift could be a result of the laws of physics being different in that distant galaxy. In this case, all attempts to reason the cause of the red-shift would be futile. There would be no basis upon which to build a theory.
I shall therefore assume the Prime Axiom to be true.
The explanation of red-shift, which requires the least number of assumptions and postulations to be stacked on top of direct observation, is that it must occur during the light's passage through the intervening space-time between source and observer. So the light emitted by the distant galaxy is not shifted in any way at source. The galaxy emits light of the same wavelengths as it would if it were local. So red-shifted is how the galaxy seems: not as it is. Consequently, the light emitted by the galaxy must become red-shifted during its journey from its distant origin. The observed red-shift cannot be due to recessional velocity or expanding space. Either would violate the Prime Axiom.
The only workable option must therefore be that the red-shift that I see is effected by the transport mechanism of space-time that conveys the light from the distant galaxy [the light-source] to me [the observer].
The Æthereal Model
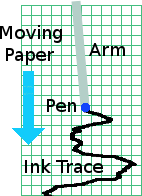 The æthereal model of the universe, which I have been building throughout this series of essays, is the way I have chosen to describe this transport mechanism of space-time by which light is conveyed from its source to an observer. The basic concept of this model, is that there is a fundamental fabric of space-time, which I have called the æther. It flows convergently into sink-holes, which constitute the cores of all primary particles of matter. The æther flows radially inwards towards a sink hole at a constant velocity of c. A light-source etches its light signature onto the passing æther in the same way that a moving pen writes an ink trace onto the passing paper of a chart recorder.
The æthereal model of the universe, which I have been building throughout this series of essays, is the way I have chosen to describe this transport mechanism of space-time by which light is conveyed from its source to an observer. The basic concept of this model, is that there is a fundamental fabric of space-time, which I have called the æther. It flows convergently into sink-holes, which constitute the cores of all primary particles of matter. The æther flows radially inwards towards a sink hole at a constant velocity of c. A light-source etches its light signature onto the passing æther in the same way that a moving pen writes an ink trace onto the passing paper of a chart recorder.
If the Prime Axiom be true, then atoms of a particular kind, under the same conditions, are everywhere the same size and change state at the same rate. Consequently, their corresponding spectral lines will each be of the same wavelength and frequency. In other words, the spectral lines emitted by atoms of a particular type will be coincident, wherever in the universe those atoms may be. So provided the æther does not stretch radially during its convergent journey from the edge of my event horizon, then I should not see any distant galaxy as red-shifted.
Yet I do. I therefore deduce that, during its long journey from a distant galaxy at the edge of my event-horizon, the æther, which is flowing into each sink-hole within the atoms in the retinas of my eyes, must, by the time it reaches me, have stretched radially to 7 times what it was when it passed by the source. For this to happen, the æther, as it reaches me, must be moving at 7 times the velocity at which it was moving when it passed the source. Thus, the æthereal flux appears to have a terminal inward radial velocity of 'c' as it enters a sink hole, which diminishes with radial distance from the sink hole.
But why should it? I see two related æthereal variables at play here; namely, it's flux density and its velocity of convergence. As evinced by pure spherical geometry, the æthereal flux density increases with decreasing radial distance from a sink hole at a rate of 8πr, where 'r' is the distance from the sink hole. If the æther be a self-influencing fluid, then its density will increase slightly faster with decreasing radial distance than what would be due to pure spherical geometry. In other words, it would increase in density at a rate of k×8πr, where 'k' is a very small constant with a value somewhere of the order of the Hubble 'constant' divided by 8π.
This is consistent with the behaviour of a material fluid such as the 'perfect' gas [with non-massless molecules] flowing spherically radially into a point sink. Notwithstanding, one must remember, when considering the æther, that it is not a material fluid but a velocity-fluid: it exists, from any observer's point of view, only so long as he be in forced motion relative to it. Consequently, its behaviour follows rules that are one order of differentiation less than those for material fluids. Its rate of change of density with decreasing radial distance would therefore not be k×8πr but simply k×8π. It would be linear.
Suppose that the governing criterion be that the æthereal flux enter a sink hole at a universally constant terminal velocity 'c'. Since light arriving from very distant sources is red-shifted, the æthereal 'chart roll' on which such sources etch their light signatures must have passed those sources at velocities much less than 'c'. The only remaining questions concern the manner in which the velocity of the æthereal essence diminishes with radial distance from its destination sink hole. Does it diminish linearly or non-linearly? If non-linearly, does it diminish more rapidly or less rapidly with increasing distance?
The problem with these questions is the notion of distance. They promote distance as the fundament against which a judgement about velocity is made. But in the context of the universe, it is motion that is the fundament, of which distance is merely the special case of zero relative motion.
In our terrestrial environment, which is the cradle of our conceptual understanding of the physical domain, distance is the basis in terms of which we judge all physical form and behaviour. Notwithstanding, our terrestrial environment is only a very limited special case of universal reality. In the universe as a whole, the fundamental notion is 'free-fall' relative motion. In other words, the ground state of the universe is where objects are moving relative to each other while free of the influence of any deliberate external forces. Such motion can be complex. It can involve highly non-linear relative accelerations as well as constant relative velocities.
A corollary to this is that there can be no real difference between a galaxy receding from an observer as a result of it travelling through space at a particular velocity of recession or as a result of the space in between them expanding at the same rate.
The question therefore should not be about how non-linear the diminution in the velocity of the æthereal essence with radial distance may be. It would be more appropriate to regard the diminution in the velocity of the æthereal essence with radial distance as linear and apply a compensatory non-linearity to our perception of galactic distances.
Please note that it is the velocity of my inflowing æthereal essence that linearly diminishes with radial distance from me. Thus it is only my inflowing æthereal essence that is passing a far galaxy slowly enough to cause the amount of red-shift I see. The velocity with which the æthereal essence is entering sink holes within that far galaxy is still 'c': the same velocity with which it enters the sink holes in the constituents of the atoms in the retinas of my eyes. What happens in both places is the same. The Prime Axiom is thereby conserved.
It is clear that, within the context of my æther-based theory of the universe, the rate at which the radial velocity of the 3-D æthereal conveyor diminishes must be exceedingly small. I would expect it to be of a similar value to that of the Hubble 'constant'; namely, of the order of seventy-odd kilometres per second per mega-parsec. Consequently, there must be a threshold radial distance from me at which the radial velocity of my æthereal in-flow becomes too slow for an atom to be able to inscribe upon it an 'electromagnetic' [or, in my æthereal view, an 'inertial'] etching with adequate depth of modulation or clarity of form to be observable. And that threshold radial distance is what I would say marks the edge of my event-horizon.
In any case, at such an extreme distance, the red-shift is so great that 'electromagnetic' waves are too long to resolve any detail about their source. Thus, what I observe as the Cosmic Microwave Background is the fuzzy last frontier of my event-horizon at which anything at all can be discerned. But this does not mean that the universe does not continue beyond my event-horizon. I would expect it to continue, just as does the surface of the earth beyond my terrestrial horizon. Furthermore, I see no reason why it must not continue in the same form. That is, with the same kinds of planets, stars and galaxies as are observed in my cosmic proximity.
In constructing this theory of red-shift, I committed what is perhaps the ultimate scientific blasphemy. I have proposed that the universal æther, upon which a distant source etches its light signature, does not flow towards me at a constant radial velocity. To explain the phenomenon of red-shift, I have proposed that it flows slowly from the depths of space, increasing linearly up to a terminal velocity of 'c' as it enters the sink holes in the atoms of the retinas of my eyes. This is effectively saying that the velocity of light is not a constant. But the constancy of the velocity of light is the prime anchor of practically all modern scientific theories. So what does science know about the velocity of light. Within what scope of cosmic bounds has it been verified as constant?
Science has only measured the velocity of light within what is, cosmically speaking, a minuscule context of about 2 milli-light-years. That is the latest [at the time of writing] two-way radio exchange [or active radar verification] between Earth and Voyager I. To say that ipso facto the velocity of light, from the point of view of any particular observer, must therefore be constant over a 13 billion light-year path from the edge of his event-horizon is just not credible, especially considering the extremely small rate of diminution required to explain red-shift.
Please continue to remember that my proposed idea that the radial velocity of the æthereal in-flow to an observer is relativistic. It is the same for any observer anywhere in the universe. An observer at the edge of our current event-horizon will perceive our Milky Way galaxy to be red-shifted. Spectral lines from sources within his vicinity will appear to him as the spectral lines emitted by sources in our vicinity do to us. The laws of physics are the same everywhere. The Prime Axiom is conserved.
Conclusion
My æthereal in-flow model is, like any other scientific theory, nothing more than a mental framework with which I try to get a grasp on reality. It is however — at least to me — a much simpler explanation than any nebulous notions of expanding space. So the distant galaxies may not be moving away after all. They could, in fact, be approaching us. Just the way local celestial objects do with their decaying orbits.
© 17 July 2018 Robert John Morton |
PREV |
NEXT

 Following the advent of powerful astronomical telescopes, observers saw objects much further out into space than had been previously possible. These newly-sighted objects looked redder than closer stars and galaxies that had been known for millennia. At first, it seemed that the bluer colours were being filtered out, more than were the redder ones, by the greater amount of interstellar medium through which light from these new more distant objects had to pass. But it was not that simple. This is because atomic emission and absorption lines within the light from these new more distant objects were also shifted towards the redder end of the visible spectrum. The wavelength of the light received must therefore necessarily have become greater than it was when it left its source.
Following the advent of powerful astronomical telescopes, observers saw objects much further out into space than had been previously possible. These newly-sighted objects looked redder than closer stars and galaxies that had been known for millennia. At first, it seemed that the bluer colours were being filtered out, more than were the redder ones, by the greater amount of interstellar medium through which light from these new more distant objects had to pass. But it was not that simple. This is because atomic emission and absorption lines within the light from these new more distant objects were also shifted towards the redder end of the visible spectrum. The wavelength of the light received must therefore necessarily have become greater than it was when it left its source.
 Depicted within science's view of 4-dimensional space-time, all events, whose influence can affect me, at the moment we call now, must lie on the infinitely thin surface of the infinite red cone, as shown in the adjacent diagram. Such an event is indicated by the figure (1). An event inside the cone (2) happened too close to me for me to be affected by it now. Its influence passed by my present position sometime in the past and is currently travelling away from me. I may have been affected by it in the past, so long as its influence passed my location after I was born. An event outside the cone (3) happened too far away from me for its influence to have yet reached my current location. I could be affected by it in the future if I am still alive when its effect reaches me.
Depicted within science's view of 4-dimensional space-time, all events, whose influence can affect me, at the moment we call now, must lie on the infinitely thin surface of the infinite red cone, as shown in the adjacent diagram. Such an event is indicated by the figure (1). An event inside the cone (2) happened too close to me for me to be affected by it now. Its influence passed by my present position sometime in the past and is currently travelling away from me. I may have been affected by it in the past, so long as its influence passed my location after I was born. An event outside the cone (3) happened too far away from me for its influence to have yet reached my current location. I could be affected by it in the future if I am still alive when its effect reaches me.
 The diagram on the right shows the situation at an arbitrary instant called 'now'. The events E1, E2 and E3 occur simultaneously in time but at different distances from me. The radial in-flux of the æther brings information from each of these 3 events radially towards me at the velocity of light. I cannot see any evidence at this moment that event E1 ever occurred. This is because event E1 occurred too close to me. So all information about its occurrence will have passed the point where I am 'now' at some time in my past. I may have seen it in my past, assuming it passed by me after I was born. But I cannot see it now, nor will I ever see it in my future.
The diagram on the right shows the situation at an arbitrary instant called 'now'. The events E1, E2 and E3 occur simultaneously in time but at different distances from me. The radial in-flux of the æther brings information from each of these 3 events radially towards me at the velocity of light. I cannot see any evidence at this moment that event E1 ever occurred. This is because event E1 occurred too close to me. So all information about its occurrence will have passed the point where I am 'now' at some time in my past. I may have seen it in my past, assuming it passed by me after I was born. But I cannot see it now, nor will I ever see it in my future.
 The diagram on the left again shows the situation at an arbitrary instant called 'now'. Here, however, events E1, E2 and E3 occur at places that are equally distant from me but at different times. The radial in-flux of the æther brings information from each of these 3 events radially towards me at the velocity of light. I cannot see any evidence at this moment that event E1 ever occurred. This is because event E1 occurred too late [too close to me in time]. So all information about its occurrence will have passed the point where I am 'now' at some time in my past. I may have seen it in my past, assuming it passed by me after I was born. But I cannot see it now, nor will I ever see it in my future.
The diagram on the left again shows the situation at an arbitrary instant called 'now'. Here, however, events E1, E2 and E3 occur at places that are equally distant from me but at different times. The radial in-flux of the æther brings information from each of these 3 events radially towards me at the velocity of light. I cannot see any evidence at this moment that event E1 ever occurred. This is because event E1 occurred too late [too close to me in time]. So all information about its occurrence will have passed the point where I am 'now' at some time in my past. I may have seen it in my past, assuming it passed by me after I was born. But I cannot see it now, nor will I ever see it in my future.
 The universe that I see 'now' is illustrated in the diagram on the right. The condition that an event be included within my event-horizon is that information about it is just at the point of arriving at the place where I am now. Such events do not have to be simultaneous from my point of view, nor do they need to be equidistant from me. They each simply need to fulfil the condition: r = c × t, viz:
The universe that I see 'now' is illustrated in the diagram on the right. The condition that an event be included within my event-horizon is that information about it is just at the point of arriving at the place where I am now. Such events do not have to be simultaneous from my point of view, nor do they need to be equidistant from me. They each simply need to fulfil the condition: r = c × t, viz:
 In the diagram on the left, the small central blue sphere represents the limit at which stellar distances can be determined geometrically to an accuracy of ±10%. Its radius is about 4 millimetres on my computer screen. On this same scale, the brown circle representing the source of the Cosmic Microwave Background should really have a radius of 110 kilometres. In other words, almost 28 million times the maximum distance at which it is possible to measure stellar distances accurately by parallax. Can it be safe to assume that, what appears to be ostensibly linear throughout its range of measurability, will remain so when it is extrapolated to 28 million times that range?
In the diagram on the left, the small central blue sphere represents the limit at which stellar distances can be determined geometrically to an accuracy of ±10%. Its radius is about 4 millimetres on my computer screen. On this same scale, the brown circle representing the source of the Cosmic Microwave Background should really have a radius of 110 kilometres. In other words, almost 28 million times the maximum distance at which it is possible to measure stellar distances accurately by parallax. Can it be safe to assume that, what appears to be ostensibly linear throughout its range of measurability, will remain so when it is extrapolated to 28 million times that range?
 To get a sense of scale here, let us consider our own local galaxy, the Milky Way. We are inside the Milky Way galaxy, located approximately where the red circle is as shown on the right. The diameter of our galaxy is estimated to be from 100 to 180 thousand light-years. Let us suppose it has a radius of 70,000 light-years. The maximum range out to which we can measure the distance of an object accurately by geometrical parallax is about 500 light-years. We can therefore only estimate the distance of a stellar object accurately up to 500 ÷ 70,000 ≅ 0·007 of the radius of our own galaxy. That is as far out as the radius of the white dot inside the red circle shown in the illustration.
To get a sense of scale here, let us consider our own local galaxy, the Milky Way. We are inside the Milky Way galaxy, located approximately where the red circle is as shown on the right. The diameter of our galaxy is estimated to be from 100 to 180 thousand light-years. Let us suppose it has a radius of 70,000 light-years. The maximum range out to which we can measure the distance of an object accurately by geometrical parallax is about 500 light-years. We can therefore only estimate the distance of a stellar object accurately up to 500 ÷ 70,000 ≅ 0·007 of the radius of our own galaxy. That is as far out as the radius of the white dot inside the red circle shown in the illustration.
 Here on Earth, an atom of a particular type [let’s say it's hydrogen], when provoked in some way, absorbs (1) a naturally-determined quantity of 'electromagnetic' energy of a narrow naturally determined wavelength. This jolts the atom (2) up to one of its HIGH energy states. This narrow naturally determined wavelength, at which the atom absorbs this 'electromagnetic' energy, is called an absorption line.
Here on Earth, an atom of a particular type [let’s say it's hydrogen], when provoked in some way, absorbs (1) a naturally-determined quantity of 'electromagnetic' energy of a narrow naturally determined wavelength. This jolts the atom (2) up to one of its HIGH energy states. This narrow naturally determined wavelength, at which the atom absorbs this 'electromagnetic' energy, is called an absorption line.
 The emission lines of hydrogen that fall within the visible spectrum are illustrated on the right. Their relative spacings are a unique signature showing that hydrogen is present in a source of light.
The emission lines of hydrogen that fall within the visible spectrum are illustrated on the right. Their relative spacings are a unique signature showing that hydrogen is present in a source of light.
 Or perhaps the interstellar medium, through which the light has passed, attenuates or disperses the shorter [bluer] wavelengths much more than the longer [redder] wavelengths (see illustration).
Or perhaps the interstellar medium, through which the light has passed, attenuates or disperses the shorter [bluer] wavelengths much more than the longer [redder] wavelengths (see illustration).
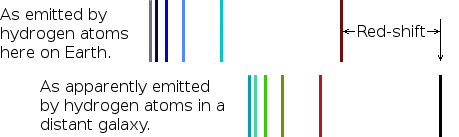
 A hydrogen atom at the far reaches of my event-horizon spontaneously emits a pulse of green light [as arrowed in the adjacent diagram]. About 12 billion years later, I see that event here on Earth.
A hydrogen atom at the far reaches of my event-horizon spontaneously emits a pulse of green light [as arrowed in the adjacent diagram]. About 12 billion years later, I see that event here on Earth.
 The æthereal model of the universe, which I have been building throughout this series of essays, is the way I have chosen to describe this transport mechanism of space-time by which light is conveyed from its source to an observer. The basic concept of this model, is that there is a fundamental fabric of space-time, which I have called the æther. It flows convergently into sink-holes, which constitute the cores of all primary particles of matter. The æther flows radially inwards towards a sink hole at a constant velocity of c. A light-source etches its light signature onto the passing æther in the same way that a moving pen writes an ink trace onto the passing paper of a chart recorder.
The æthereal model of the universe, which I have been building throughout this series of essays, is the way I have chosen to describe this transport mechanism of space-time by which light is conveyed from its source to an observer. The basic concept of this model, is that there is a fundamental fabric of space-time, which I have called the æther. It flows convergently into sink-holes, which constitute the cores of all primary particles of matter. The æther flows radially inwards towards a sink hole at a constant velocity of c. A light-source etches its light signature onto the passing æther in the same way that a moving pen writes an ink trace onto the passing paper of a chart recorder.